二维区域和检索 - 矩阵不可变

304. 二维区域和检索 - 矩阵不可变

给定一个二维矩阵 matrix，以下类型的多个请求：

计算其子矩形范围内元素的总和，该子矩阵的 左上角 为 (row1, col1) ，右下角 为 (row2, col2) 。

实现 NumMatrix 类：

NumMatrix(int[][] matrix) 给定整数矩阵 matrix 进行初始化
int sumRegion(int row1, int col1, int row2, int col2) 返回 左上角 (row1, col1) 、右下角 (row2, col2) 所描述的子矩阵的元素总和。

示例 1：

width: 200px;

输入: 
["NumMatrix","sumRegion","sumRegion","sumRegion"]
[[[[3,0,1,4,2],[5,6,3,2,1],[1,2,0,1,5],[4,1,0,1,7],[1,0,3,0,5]]],[2,1,4,3],[1,1,2,2],[1,2,2,4]]
输出: 
[null, 8, 11, 12]

解释:
NumMatrix numMatrix = new NumMatrix([[3,0,1,4,2],[5,6,3,2,1],[1,2,0,1,5],[4,1,0,1,7],[1,0,3,0,5]]);
numMatrix.sumRegion(2, 1, 4, 3); // return 8 (红色矩形框的元素总和)
numMatrix.sumRegion(1, 1, 2, 2); // return 11 (绿色矩形框的元素总和)
numMatrix.sumRegion(1, 2, 2, 4); // return 12 (蓝色矩形框的元素总和)

提示：

m == matrix.length
n == matrix[i].length
1 <= m, n <= 200
-10⁵ <= matrix[i][j] <= 10⁵
0 <= row1 <= row2 < m
0 <= col1 <= col2 < n
最多调用 10⁴ 次 sumRegion 方法

相似题目

区域和检索 - 数组不可变

二维区域和检索 - 可变

原站题解

去查看

上次编辑到这里，代码来自缓存点击恢复默认模板

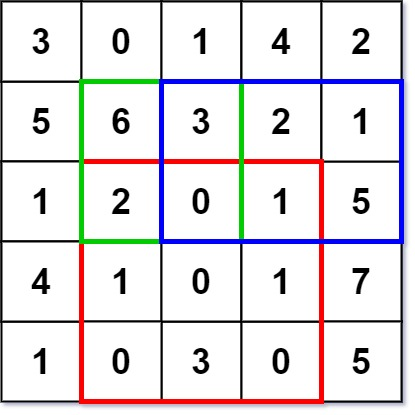
class NumMatrix { public: NumMatrix(vector<vector<int>>& matrix) { } int sumRegion(int row1, int col1, int row2, int col2) { } }; /** * Your NumMatrix object will be instantiated and called as such: * NumMatrix* obj = new NumMatrix(matrix); * int param_1 = obj->sumRegion(row1,col1,row2,col2); */

golang 解法, 执行用时: 560 ms, 内存消耗: 16.5 MB, 提交时间: 2022-11-23 11:46:24

type NumMatrix struct {
    sums [][]int
}

func Constructor(matrix [][]int) NumMatrix {
    sums := make([][]int, len(matrix))
    for i, row := range matrix {
        sums[i] = make([]int, len(row)+1)
        for j, v := range row {
            sums[i][j+1] = sums[i][j] + v
        }
    }
    return NumMatrix{sums}
}

func (nm *NumMatrix) SumRegion(row1, col1, row2, col2 int) (sum int) {
    for i := row1; i <= row2; i++ {
        sum += nm.sums[i][col2+1] - nm.sums[i][col1]
    }
    return
}

/**
 * Your NumMatrix object will be instantiated and called as such:
 * obj := Constructor(matrix);
 * param_1 := obj.SumRegion(row1,col1,row2,col2);
 */

golang 解法, 执行用时: 532 ms, 内存消耗: 16.5 MB, 提交时间: 2022-11-23 11:46:06

type NumMatrix struct {
    sums [][]int
}

func Constructor(matrix [][]int) NumMatrix {
    m := len(matrix)
    if m == 0 {
        return NumMatrix{}
    }
    n := len(matrix[0])
    sums := make([][]int, m+1)
    sums[0] = make([]int, n+1)
    for i, row := range matrix {
        sums[i+1] = make([]int, n+1)
        for j, v := range row {
            sums[i+1][j+1] = sums[i+1][j] + sums[i][j+1] - sums[i][j] + v
        }
    }
    return NumMatrix{sums}
}

func (nm *NumMatrix) SumRegion(row1, col1, row2, col2 int) int {
    return nm.sums[row2+1][col2+1] - nm.sums[row1][col2+1] - nm.sums[row2+1][col1] + nm.sums[row1][col1]
}

/**
 * Your NumMatrix object will be instantiated and called as such:
 * obj := Constructor(matrix);
 * param_1 := obj.SumRegion(row1,col1,row2,col2);
 */

python3 解法, 执行用时: 556 ms, 内存消耗: 25.7 MB, 提交时间: 2022-11-23 11:44:24

class NumMatrix:
    '''
    二维前缀和
    '''

    def __init__(self, matrix: List[List[int]]):
        m, n = len(matrix), (len(matrix[0]) if matrix else 0)
        self.sums = [[0] * (n + 1) for _ in range(m + 1)]
        _sums = self.sums

        for i in range(m):
            for j in range(n):
                _sums[i + 1][j + 1] = _sums[i][j + 1] + _sums[i + 1][j] - _sums[i][j] + matrix[i][j]

    def sumRegion(self, row1: int, col1: int, row2: int, col2: int) -> int:
        _sums = self.sums

        return _sums[row2 + 1][col2 + 1] - _sums[row1][col2 + 1] - _sums[row2 + 1][col1] + _sums[row1][col1]

# Your NumMatrix object will be instantiated and called as such:
# obj = NumMatrix(matrix)
# param_1 = obj.sumRegion(row1,col1,row2,col2)

python3 解法, 执行用时: 2064 ms, 内存消耗: 25.1 MB, 提交时间: 2022-11-23 11:43:38

class NumMatrix:
    '''
    一维前缀和
    '''
    def __init__(self, matrix: List[List[int]]):
        m, n = len(matrix), (len(matrix[0]) if matrix else 0)
        self.sums = [[0] * (n + 1) for _ in range(m)]
        _sums = self.sums

        for i in range(m):
            for j in range(n):
                _sums[i][j + 1] = _sums[i][j] + matrix[i][j]

    def sumRegion(self, row1: int, col1: int, row2: int, col2: int) -> int:
        _sums = self.sums

        total = sum(_sums[i][col2 + 1] - _sums[i][col1] for i in range(row1, row2 + 1))
        return total

# Your NumMatrix object will be instantiated and called as such:
# obj = NumMatrix(matrix)
# param_1 = obj.sumRegion(row1,col1,row2,col2)

上一题

下一题

详情